Statistical shape analysis

Statistical shape analysis is a geometrical analysis from a set of shapes in which statistics are measured to describe geometrical properties from similar shapes or different groups, for instance, the difference between male and female Gorilla skull shapes, normal and pathological bone shapes, etc. Some of the important aspects of shape analysis are to obtain a measure of distance between shapes, to estimate average shapes from a (possibly random) sample and to estimate shape variability in a sample[1]. One of the main methods used is principal component analysis.

Contents

Modeling

See also: Point distribution model

The first step after collecting a set of shapes is creating a proper shape model for further statistical analysis. A shape is determined by a finite number of coordinate points, known as landmark points; the Cartesian coordinates is the most commonly used one.

Shape deformation

In physics, deformation is a change of a shape due to an applied force. Investigating shape deformation can reveal the transformation between two similar shapes and give information about local and global shape differences. Mathematically, a deformation is defined as a mapping from a shape t to y by a transformation function Φ, i.e. 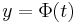y = \Phi(t) .(See Definition 10.2 of I.L. Dryden and K.V. Mardia (1998). Statistical Shape Analysis. John Wiley & Sons. ISBN 0471958166. )

See also

References

  1. ^ I.L. Dryden and K.V. Mardia (1998). Statistical Shape Analysis. John Wiley & Sons. ISBN 0471958166.